„Matemetikai inga” változatai közötti eltérés
(Új oldal, tartalma: „Szerkesztés alatt.”) |
a |
||
| (egy szerkesztő 4 közbeeső változata nincs mutatva) | |||
| 1. sor: | 1. sor: | ||
| − | + | [[Kategória:Szerkesztő:Hartlein]] | |
| + | <wikitex> | ||
| + | |||
| + | [[Fájl:Matematikai_inga.ogv|thumbtime=0:07|bélyegkép|720px|[[Matemetikai inga]]]] | ||
| + | == Az elhangzó szöveg == | ||
| + | |||
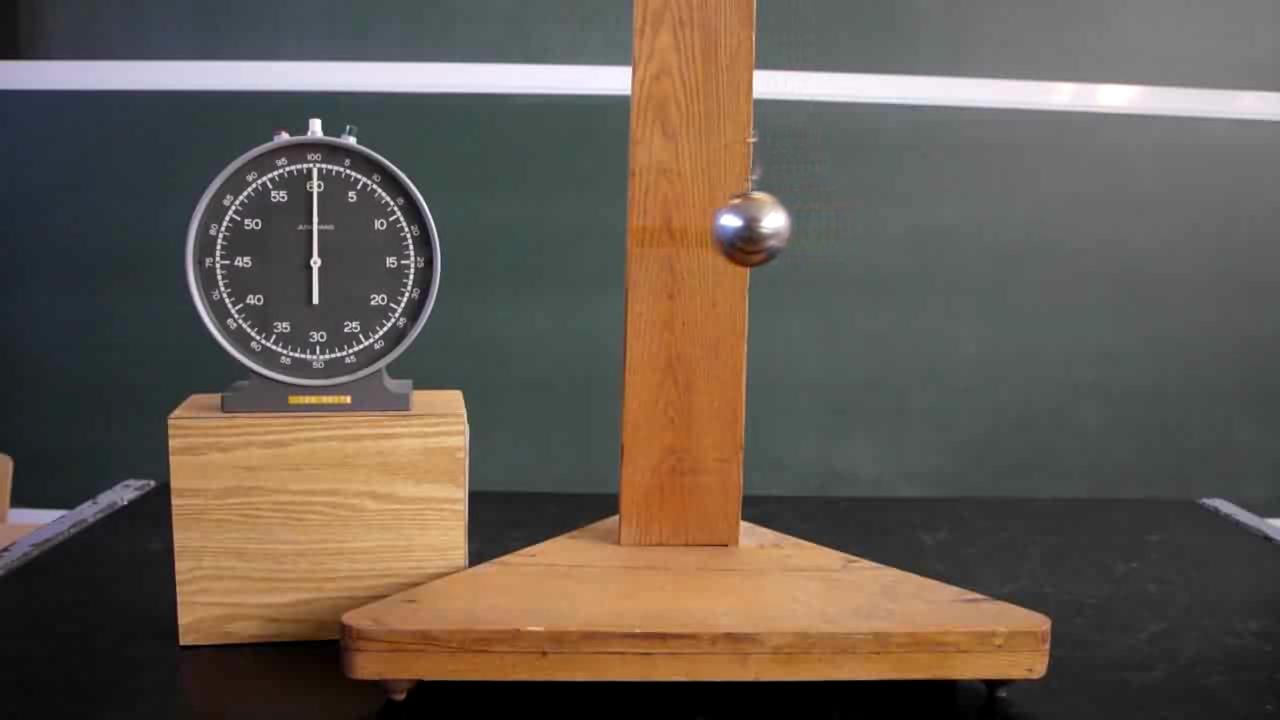
| + | A matematikai inga lengéseit egy kisméretű, nagy tömegű acélgolyó mozgása mutatja be a legeredményesebben. Ekkor mondhatjuk, hogy a lengésidő az inga hosszának négyzetgyökével arányos. | ||
| + | Adott hosszúságú ingánál mérjük meg 10 lengés idejét, majd ismételjük meg a mérést fele olyan hosszúságú ingával. A mért lengésidők hányadosának négyzete jó közelítéssel valóban kettő. | ||
| + | |||
| + | == Simple Gravity Pendulum == | ||
| + | |||
| + | The oscillations of a simple gravity pendulum can be demonstrated most effectively by the movements of a small, heavy steel ball. In this case we can say that the period is directly proportional to the square root of the length of the pendulum. | ||
| + | Let us measure 10 periods of a pendulum with known length, then let us repeat the measurement with a pendulum with half the same length. The square of the ratio of the periods will indeed be close to two. | ||
| + | |||
| + | </wikitex> | ||
A lap jelenlegi, 2014. március 9., 12:24-kori változata
Az elhangzó szöveg
A matematikai inga lengéseit egy kisméretű, nagy tömegű acélgolyó mozgása mutatja be a legeredményesebben. Ekkor mondhatjuk, hogy a lengésidő az inga hosszának négyzetgyökével arányos. Adott hosszúságú ingánál mérjük meg 10 lengés idejét, majd ismételjük meg a mérést fele olyan hosszúságú ingával. A mért lengésidők hányadosának négyzete jó közelítéssel valóban kettő.
Simple Gravity Pendulum
The oscillations of a simple gravity pendulum can be demonstrated most effectively by the movements of a small, heavy steel ball. In this case we can say that the period is directly proportional to the square root of the length of the pendulum. Let us measure 10 periods of a pendulum with known length, then let us repeat the measurement with a pendulum with half the same length. The square of the ratio of the periods will indeed be close to two.