„Spintronika” változatai közötti eltérés
(→Landauer formula spinpolarizált esetben) |
(→Landauer formula spinpolarizált esetben) |
||
| 97. sor: | 97. sor: | ||
alakba. Ez a formula lehetőséget ad arra, hogy a spinpolarizációt sávszerkezet-parameterek (Fermi-felület állapotsűrűsége és Fermi-sebesség) alapján értelmezzük. | alakba. Ez a formula lehetőséget ad arra, hogy a spinpolarizációt sávszerkezet-parameterek (Fermi-felület állapotsűrűsége és Fermi-sebesség) alapján értelmezzük. | ||
| + | Ferromágneses anyag sávmodelljét szemlélteti a 4. ábra. Mindkét panelen az energiatengelytől jobbra a fel, míg balra a le spinű elektronok állapotsűrűsége létszik. Az $s$-elektronokhoz tartozó parabolikus energiafüggésű állapotsűrűség mellett a keskeny $d$ (vagy $f$) sávokhoz tartozó állapotűrűség-csúcsokat láthatjuk, az utóbbi energiában felhasad a kicserélődési kölcsönhatás miatt. | ||
| + | Az anyag mágnesezettsége a betöltött (Fermi-energia alatti) fel és le spinű elektronok számának különbségéből adódik. Mindkét panelen a fel spinű elektronok vannak nagyobb számban, azaz a fel spint tekintjük többségi spinorientációnak. Ezzel szemben a transzport tulajdonságokhoz, így az áram spinpolarizációjához csak a Fermi-felületen közelében levő elektronok adnak járulékot. Az a) panelen a fel spinű elektronok vannak jelen nagyobb számmal a Fermi-szintnél, így pozitív a spinpolarizáció. Ezzel szemben a b) panelen a Fermi-energia máshol helyezkedik el a $d$-sávokhoz képest, így végül a le spinű elektronok állnak rendelkezésre nagyobb számban a Fermi-energiánál, azaz a pozitív mágnesezettség ellenére negatív spinpolarizációt kapunk. Negatív spinpolarizációt valós anyagokban is tapasztalhatunk, pl. Co és Ni esetében. | ||
| + | |||
| + | ==Spinpolarizált transzport alkalmazása: spin szelep== | ||
| − | + | A szabadsági fok kihasználásával számos érdekes eszközt építhetünk. A továbbiakban a gyakorlati felhasználás szempontjából legelterjedtebb, merevlemezek olvasófejében alkalmazott eszközt, a spin-szelepet mutatjuk be. | |
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | Az alap ötlet, az óriás mágneses ellenállást mutató nanoszerkezet felfedezése Albert Fert \cite{PhysRevLett.61.2472} és Peter Grünberg nevéhez kötődik, akik 2007-ben Nobel-díjat kaptak felfedezésükért. Az szerkezet két ferromágneses rétegből áll, melyeket egy, a spindiffúziós hossznál vékonyabb paramágneses réteg köt öszze. A tapasztalatok alapján ennek a nanostruktúrának az ellenállása lényegesen nagyobb akkor, ha a két mágneses réteg mágnesezettsége azonos irányba mutat, mint ha ellentétes irányba mutatnak. Ezt a jelenséget lehet kihasználni mágnesesen tárolt információ kiolvasására, ha a felső réteg mágnesezettségét rögzítjuk, az alsóréteg mágnesezettsége pedig az alatta mozgatott adattároló lemez mágnesezettségének megfelelően áll be, így az információ egyszerű mágnesezettség méréssel kiolvasható. Ezzel a módszerrel a merevlemezek tárolókapacitásának jelentős növekedését lehetett elérni az eredeti induktív, illetve a későbbi anizotróp mágneses ellenállás mérésen alapuló módszerekhez képest. | |
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
{| cellpadding="5" cellspacing="0" align="center" | {| cellpadding="5" cellspacing="0" align="center" | ||
| 161. sor: | 113. sor: | ||
|} | |} | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
To understand this phenomenon, as the simplest model we can consider an ideal | To understand this phenomenon, as the simplest model we can consider an ideal | ||
A lap 2013. július 1., 10:10-kori változata
Spindiffúziós hossz
Egy makroszkopikus vezetőben az elektronok számtalanszor szóródnak miközben eljutnak eaz egyik elektródából a másikba. Ahogy a nanovezetékek tárgyalásának bevezetésekor láttuk a szennyezőkön és rácshibákon történő rugalmas szórás az elektronok elektromos tértől nyert impulzusának elvesztéséhez vezet. Ennek a folyamatnak a karakterisztikus skáláját az átlagos momentumrelaxációs szabadúthossz, 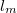 jellemzi. Rugalmatlan szórások esetén (pl. kölcsönhatás rácsrezgésekkel) az elektronok energiája megváltozik, és így elveszik a a fázisinformáció, azaz megszűnik az interferenciaképesség. Ennek a folyamatnak a karakterisztikus skálája az ún. fázisdiffúziós szabadúthossz,
jellemzi. Rugalmatlan szórások esetén (pl. kölcsönhatás rácsrezgésekkel) az elektronok energiája megváltozik, és így elveszik a a fázisinformáció, azaz megszűnik az interferenciaképesség. Ennek a folyamatnak a karakterisztikus skálája az ún. fázisdiffúziós szabadúthossz, 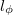 . Megfelelő távolságon belül az elektronok a mágneses momentumuk, azaz a spinjükhöz tartozó információt is elvesztik, amit a spindiffúziós hosszal,
. Megfelelő távolságon belül az elektronok a mágneses momentumuk, azaz a spinjükhöz tartozó információt is elvesztik, amit a spindiffúziós hosszal, 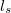 jellemezhetünk.
Egy spindiffúziós hossznál kisebb, mágnesesen rendezett építőelemeket tartalmazó nanoszerkezetben azonban számos érdekes, az elektronok spin szerinti polarizáltságához kötődő jelenséggel találkozhatunk.
jellemezhetünk.
Egy spindiffúziós hossznál kisebb, mágnesesen rendezett építőelemeket tartalmazó nanoszerkezetben azonban számos érdekes, az elektronok spin szerinti polarizáltságához kötődő jelenséggel találkozhatunk.
Spinpolarizáció ideális nanovezetékekben
A korábbiakban láttuk, hogy ha két elektróda közé egy ideális, szórásmentes nanovezetéket helyezünk, akkor abban keresztirányban állóhullámok, hosszirányban pedig síkhullám terjedés alakul ki, a vezetőképesség pedig 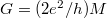 , ahol M a nyitott vezetési csatornák száma, azaz azon különböző keresztmódusokhoz tartozó egydimenziós parabolikus diszperziók száma, melyek metszik a Fermi-energiát. A kettes szorzó a spin szerinti degenerációból adódott.
, ahol M a nyitott vezetési csatornák száma, azaz azon különböző keresztmódusokhoz tartozó egydimenziós parabolikus diszperziók száma, melyek metszik a Fermi-energiát. A kettes szorzó a spin szerinti degenerációból adódott.
Egy ferromágneses nanovezetékben azonban különbséget kell tenni a fel és a le spinű elektronok között.
A vezeték mágnesezettségét legegyszerűbben Stoner-képben vehetjük figyelembe, azaz az energidiszperziókhoz hozzáadjuk a kicserőlédési energiát, mely 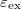 -el különbözik a fel illetve le spinű (
-el különbözik a fel illetve le spinű (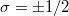 ) elektronokra:
) elektronokra:
![\[\varepsilon_n^{\sigma}(k)=\varepsilon(k)+\varepsilon_n-\sigma\varepsilon_{\mathrm{ex}}.\]](/images/math/1/e/c/1ec90d32189d7f827dd5dcf27b159654.png)
Emlékeztetőül: 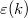 a hosszirányú terjedést,
a hosszirányú terjedést, 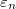 pedig a keresztirányú állóhullámok energiáját írja le. Fontos megjegyezni, hogy
pedig a keresztirányú állóhullámok energiáját írja le. Fontos megjegyezni, hogy 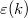 lehet tetszőleges Bloch-állapot diszperziója, nem kell feltétlenül szabad elektronoknak megfelelő parabolikus diszperziót feltételezni.
lehet tetszőleges Bloch-állapot diszperziója, nem kell feltétlenül szabad elektronoknak megfelelő parabolikus diszperziót feltételezni.
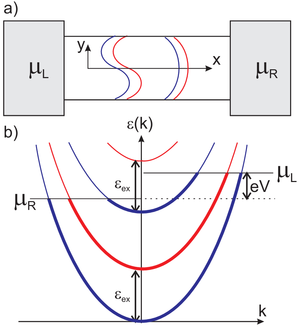
Az 1. ábra parabolikus szabad elektron diszperzió esetén szemlélteti az energiaviszonyokat. A kék görbék a fel, a piros görbék pedig a le spinű elektronokhoz tartoznak, azaz a piros parabolák minig 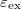 energiával a megfelelő kék parabola felett helyezkednek el. A korábbiaknak megfelelően a
energiával a megfelelő kék parabola felett helyezkednek el. A korábbiaknak megfelelően a 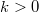 állapotok a bal oldali eletródából származnak, így annak a
állapotok a bal oldali eletródából származnak, így annak a 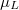 kémiai potenciákjáig vannak betöltve, míg a
kémiai potenciákjáig vannak betöltve, míg a  állapotok a jobb oldali,
állapotok a jobb oldali, 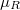 kémiai potenciálú eletródából származnak.
kémiai potenciálú eletródából származnak.
| 1. ábra |
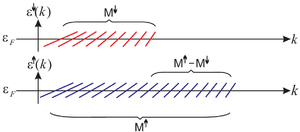
Értelemszerűen egy ferromágneses nanovezetékben a nyitott csatornák száma különbözhet a különböző spinű elektronokra (2. ábra), így az áram
![\[ I=\sum_{\sigma =\frac{1}{2},-\frac{1}{2}} \frac{e^2}{h}M^{\sigma}V \]](/images/math/2/b/7/2b7d2e5ba1e46a9261434fa189e97c4c.png)
Alakban írható, ahol 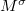 (illetve máshogy jelölve
(illetve máshogy jelölve 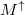 és
és 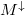 ) a fel és le spinű elektronokhoz tartozó nyitott csatornák száma.
) a fel és le spinű elektronokhoz tartozó nyitott csatornák száma.
| 2. ábra. Diszperziós görbék ferromágneses nanovezetékben a Fermi-energia körül. Fel spinű elektronoknak (kék) több nyitott vezetési csatorna áll rendelkezésre mint le spinű elektronoknak (piros). |
Fontos megjegyezni, hogy itt figyelembe vettük, hogy a nanovezetéken belül az elektronok spinállapota nem változik, ennek köszönhető hogy a fel és le spinű elektronokat egymástól független csatornaként kezelhetjük az áram felírásakor.
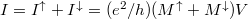 . Látjuk, hogy ferromágneses ideális nanovezetékben a vezetőképesség
. Látjuk, hogy ferromágneses ideális nanovezetékben a vezetőképesség  szerint kvantált.
szerint kvantált.
Az elektronok spin szerinti polarizáltságának fokát
![\[ P_c=\frac{I^{\uparrow}-I^{\downarrow}}{I^{\uparrow}+I^{\downarrow}} \]](/images/math/0/e/5/0e5488e876f0c988e1bdb1710aabad98.png)
képlettel definiálhatjuk, ami ideális nanovezetékben 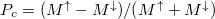 formában is írható. Ez a spinpolarizáció
formában is írható. Ez a spinpolarizáció 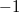 és
és 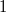 közötti értékeket vehet föl, tökéletes spinpolarizáció (
közötti értékeket vehet föl, tökéletes spinpolarizáció ( ) félfémben érhető el, amikor csak fel spinű elektronok találhatók a Fermi-energia környékén. Fontos megjegyezni, hogy mindig a fel spint vesszük többségi spinorientációnak, mely a ferromágneses tartomány mágnesezettség-irányának felel meg. Később látni fogjuk, hogy a spinpolarizáció lehet a mágnesezettséggel ellentétes előjelű.
) félfémben érhető el, amikor csak fel spinű elektronok találhatók a Fermi-energia környékén. Fontos megjegyezni, hogy mindig a fel spint vesszük többségi spinorientációnak, mely a ferromágneses tartomány mágnesezettség-irányának felel meg. Később látni fogjuk, hogy a spinpolarizáció lehet a mágnesezettséggel ellentétes előjelű.
Landauer formula spinpolarizált esetben
Egy mágneses nanovezeték valósághűbb leírását kapjuk, ha szórást is megengedünk a vezetéken belül. Ezt a legegyszerűbben a korábban megismert Landauer formalizmus keretében tehetjük meg. A Landauer képben az elektrontranszportot 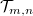 transzmisszós valószínűségekkel írjuk le, melyek a bal oldali
transzmisszós valószínűségekkel írjuk le, melyek a bal oldali 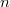 -edik vezetési csatornából a jobb oldali
-edik vezetési csatornából a jobb oldali 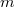 -edik csatornába történő szóródás valószínűségét adják meg. Míg
-edik csatornába történő szóródás valószínűségét adják meg. Míg 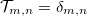 a fent tárgyalt ideális kvantumvezetéknek felel meg. Ha a vezetéken belül az elektronok rácshibákon, illetve szennyezőkön szóródnak, akkor
a fent tárgyalt ideális kvantumvezetéknek felel meg. Ha a vezetéken belül az elektronok rácshibákon, illetve szennyezőkön szóródnak, akkor 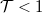 transzmissziós valószínűségeket kapunk. Fontos megjegyezni, hogy a Landauer-kép inelasztikus szórásokat (pl. elektron-fonon kölcsönhatás) és spinszórásokat nem tud figyelembe venni.
transzmissziós valószínűségeket kapunk. Fontos megjegyezni, hogy a Landauer-kép inelasztikus szórásokat (pl. elektron-fonon kölcsönhatás) és spinszórásokat nem tud figyelembe venni.
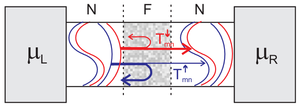
Vizsgáljuk meg a 3. ábrán szemléltetett rendszer vezetési tulajdonságait: a nanovezeték két nemmágneses ideális kvantumvezeték között egy ferromágneses tartományt tartalmaz, melyben szóródhatnak az elektronok.
| 3. ábra |
Mivel spinszórás nem történik, az áramot továbbra is egymástól függetlenül számolhatjuk a két spincsatornára a transzmissziós valószínűségek figyelembe vételével:
![\[ I^\sigma=\frac{e^2}{h}\sum_{n,m=1}^{M^\sigma}T_{m,n}^\sigma V. \]](/images/math/b/2/9/b2946c7ec106adeeaa500e7cdca002d2.png)
A 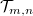 értékek függnek az elektronok Fermi-hullámhosszától, ami egy adott
értékek függnek az elektronok Fermi-hullámhosszától, ami egy adott 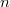 -edik csatorna esetén jelentősen eltérhet a két spin-orientációra a kicserélődési felhasadás miatt. Ez alapján spinszórás (pl. spin-pálya kölcsönhatás) nélkül is a transzmisszós valószínűségek különböznek a két spincsatornára, ezt vesszük figyelembe a
-edik csatorna esetén jelentősen eltérhet a két spin-orientációra a kicserélődési felhasadás miatt. Ez alapján spinszórás (pl. spin-pálya kölcsönhatás) nélkül is a transzmisszós valószínűségek különböznek a két spincsatornára, ezt vesszük figyelembe a  indexszel.
indexszel.
A spinfüggő áramot átírhatjuk
![\[ I^\sigma=\frac{e^2}{h}M^\sigma \bar{T}^\sigma V \]](/images/math/d/2/d/d2d35eeca080566f9f4c66f8bf6956c4.png)
formába, ahol 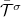 az átlagos transzmissziós valószínűség a
az átlagos transzmissziós valószínűség a  spin-orientációhoz tartozó összes nyitott vezetési csatornára. Ezzel a jelöléssel az áram spin szerinti polarizáltságát
spin-orientációhoz tartozó összes nyitott vezetési csatornára. Ezzel a jelöléssel az áram spin szerinti polarizáltságát
![\[ P_c=\frac{I^{\uparrow}-I^{\downarrow}}{I^{\uparrow}+I^{\downarrow}}=\frac{M^{\uparrow}\bar{T}^\uparrow-M^{\downarrow}\bar{T}^\downarrow}{M^{\uparrow}\bar{T}^\uparrow+M^{\downarrow}\bar{T}^\downarrow}. \]](/images/math/e/e/f/eef78545d0cb4c40985d9947b3b4657e.png)
formában írhatjuk. Spinpolarizált áramot a két spincsatorna között a nyitott vezetési csatornák számában illetve az az átlagos transzmissziós valószínűségben fellépő különbség egyaránt eredményezhet.
Kevés vezetési csatorna (pl. egyatomos kontaktus) esetén a 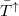 és
és 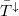 jelentős spinpolaizációt okozhat akkor is, ha a vezetési csatornák száma megegyezik a két spinirányra. Sok csatornás struktúrákban viszont
jelentős spinpolaizációt okozhat akkor is, ha a vezetési csatornák száma megegyezik a két spinirányra. Sok csatornás struktúrákban viszont 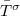 sok, különböző Fermi-hullámszámú csatornára vett átlag, így a spindiffúziós hossznál kisebb struktúrákban, ahol csak a rugalmas szórásokat vesszük figyelembe, azt átlagos transzmisszós valószínűséget közel azonosnak várjuk a két spinirányra. Ennek megfelelően
az áram spinpolarizációjához az elsődleges járulékot a vezetési csatornák számának különbsége adja:
sok, különböző Fermi-hullámszámú csatornára vett átlag, így a spindiffúziós hossznál kisebb struktúrákban, ahol csak a rugalmas szórásokat vesszük figyelembe, azt átlagos transzmisszós valószínűséget közel azonosnak várjuk a két spinirányra. Ennek megfelelően
az áram spinpolarizációjához az elsődleges járulékot a vezetési csatornák számának különbsége adja:
![\[ P_c\approx \frac{M^{\uparrow}-M^{\downarrow}}{M^{\uparrow}+M^{\downarrow}}, \]](/images/math/6/1/1/611330a8e456659423f82d2fa3ef19bc.png)
akkor is ha nem ideális a vezeték, azaz  .
.
Korábbi megfontolások alapján a vezetési csatornák számát formálisan:
![\[ M^\sigma=\frac{2\pi\hbar}{L}\sum_{n=1}^{M^\sigma}v_n^{\sigma}g_n^{\sigma}. \]](/images/math/1/4/4/144e6325977480a78786c18fdfefa79f.png)
alakban írhatjuk. Vezessünk be egy átlagos Fermi-sebességet, mely a különböző csatornák Fermi sebességeinek átlaga a csatornák állapotsűrűségével súlyozva,
![\[ \bar{v}_F^\sigma=\frac{\sum_{n} g_n^\sigma v_n^\sigma}{\sum_{n} g_n^\sigma}. \]](/images/math/7/f/d/7fdffe40d2405e6b6303c04984afd354.png)
Figyelembe véve, hogy  a Fermi szint teljes állapotsűrűsége, az áram spinpolarizációjára vonatkozó formulánkat átírhatjuk
a Fermi szint teljes állapotsűrűsége, az áram spinpolarizációjára vonatkozó formulánkat átírhatjuk
![\[ P_c\approx\frac{M^{\uparrow}-M^{\downarrow}}{M^{\uparrow}+M^{\downarrow}}=\frac{g_F^{\uparrow}\bar{v}_F^{\uparrow}-g_F^{\downarrow}\bar{v}_F^{\downarrow}}{g_F^{\uparrow}\bar{v}_F^{\uparrow}+g_F^{\downarrow}\bar{v}_F^{\downarrow}}. \]](/images/math/7/f/8/7f84d7e5b0af9c5d7c274bf6cad4e433.png)
alakba. Ez a formula lehetőséget ad arra, hogy a spinpolarizációt sávszerkezet-parameterek (Fermi-felület állapotsűrűsége és Fermi-sebesség) alapján értelmezzük.
Ferromágneses anyag sávmodelljét szemlélteti a 4. ábra. Mindkét panelen az energiatengelytől jobbra a fel, míg balra a le spinű elektronok állapotsűrűsége létszik. Az  -elektronokhoz tartozó parabolikus energiafüggésű állapotsűrűség mellett a keskeny
-elektronokhoz tartozó parabolikus energiafüggésű állapotsűrűség mellett a keskeny  (vagy
(vagy 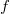 ) sávokhoz tartozó állapotűrűség-csúcsokat láthatjuk, az utóbbi energiában felhasad a kicserélődési kölcsönhatás miatt.
Az anyag mágnesezettsége a betöltött (Fermi-energia alatti) fel és le spinű elektronok számának különbségéből adódik. Mindkét panelen a fel spinű elektronok vannak nagyobb számban, azaz a fel spint tekintjük többségi spinorientációnak. Ezzel szemben a transzport tulajdonságokhoz, így az áram spinpolarizációjához csak a Fermi-felületen közelében levő elektronok adnak járulékot. Az a) panelen a fel spinű elektronok vannak jelen nagyobb számmal a Fermi-szintnél, így pozitív a spinpolarizáció. Ezzel szemben a b) panelen a Fermi-energia máshol helyezkedik el a
) sávokhoz tartozó állapotűrűség-csúcsokat láthatjuk, az utóbbi energiában felhasad a kicserélődési kölcsönhatás miatt.
Az anyag mágnesezettsége a betöltött (Fermi-energia alatti) fel és le spinű elektronok számának különbségéből adódik. Mindkét panelen a fel spinű elektronok vannak nagyobb számban, azaz a fel spint tekintjük többségi spinorientációnak. Ezzel szemben a transzport tulajdonságokhoz, így az áram spinpolarizációjához csak a Fermi-felületen közelében levő elektronok adnak járulékot. Az a) panelen a fel spinű elektronok vannak jelen nagyobb számmal a Fermi-szintnél, így pozitív a spinpolarizáció. Ezzel szemben a b) panelen a Fermi-energia máshol helyezkedik el a  -sávokhoz képest, így végül a le spinű elektronok állnak rendelkezésre nagyobb számban a Fermi-energiánál, azaz a pozitív mágnesezettség ellenére negatív spinpolarizációt kapunk. Negatív spinpolarizációt valós anyagokban is tapasztalhatunk, pl. Co és Ni esetében.
-sávokhoz képest, így végül a le spinű elektronok állnak rendelkezésre nagyobb számban a Fermi-energiánál, azaz a pozitív mágnesezettség ellenére negatív spinpolarizációt kapunk. Negatív spinpolarizációt valós anyagokban is tapasztalhatunk, pl. Co és Ni esetében.
Spinpolarizált transzport alkalmazása: spin szelep
A szabadsági fok kihasználásával számos érdekes eszközt építhetünk. A továbbiakban a gyakorlati felhasználás szempontjából legelterjedtebb, merevlemezek olvasófejében alkalmazott eszközt, a spin-szelepet mutatjuk be.
Az alap ötlet, az óriás mágneses ellenállást mutató nanoszerkezet felfedezése Albert Fert \cite{PhysRevLett.61.2472} és Peter Grünberg nevéhez kötődik, akik 2007-ben Nobel-díjat kaptak felfedezésükért. Az szerkezet két ferromágneses rétegből áll, melyeket egy, a spindiffúziós hossznál vékonyabb paramágneses réteg köt öszze. A tapasztalatok alapján ennek a nanostruktúrának az ellenállása lényegesen nagyobb akkor, ha a két mágneses réteg mágnesezettsége azonos irányba mutat, mint ha ellentétes irányba mutatnak. Ezt a jelenséget lehet kihasználni mágnesesen tárolt információ kiolvasására, ha a felső réteg mágnesezettségét rögzítjuk, az alsóréteg mágnesezettsége pedig az alatta mozgatott adattároló lemez mágnesezettségének megfelelően áll be, így az információ egyszerű mágnesezettség méréssel kiolvasható. Ezzel a módszerrel a merevlemezek tárolókapacitásának jelentős növekedését lehetett elérni az eredeti induktív, illetve a későbbi anizotróp mágneses ellenállás mérésen alapuló módszerekhez képest.
| 5. ábra |
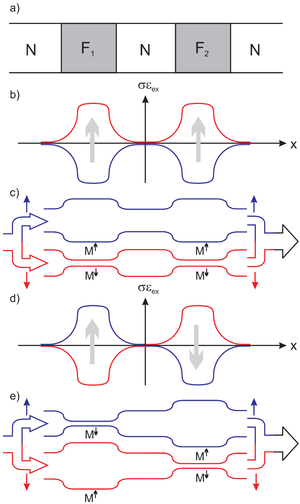
To understand this phenomenon, as the simplest model we can consider an ideal
quantum wire, in which the exchange coupling is switched on adiabatically in two
regions (Fig.~\ref{GMR1}(b,d)). The parallel orientation is modeled by a
positive exchange in both regions, whereas the antiparallel orientation
corresponds to opposite signs of the exchange in the two regions. Similarly to
the previous situations the current can be divided to two independent components
corresponding to spin up and spin down electrons. For a given spin orientation
the effect of the exchange energy is very similar to an effective spatial
narrowing or widening of the channel, which would increase/decrease the
transverse quantized energies (Fig.~\ref{GMR1}(c,e)). Therefore the system can
be considered as serial connected quantum point contacts. For electrons with
majority spin direction (spin direction parallel to the magnetization direction)
the number of open channels is denoted by 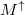 , whereas for minority
spins (antiparallel spin direction to the magnetization direction) the number of
open channels is
, whereas for minority
spins (antiparallel spin direction to the magnetization direction) the number of
open channels is 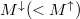 . (Note, that here the superscript
. (Note, that here the superscript
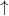 denotes majority orientation instead of the real spin direction,
and the number of open channels is also
denotes majority orientation instead of the real spin direction,
and the number of open channels is also 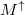 for down spins in down
oriented magnetization of the magnetic layer.)
for down spins in down
oriented magnetization of the magnetic layer.)
In such ballistic quantum point contacts the conductance is determined by the
number of modes at the narrowest cross section. If the two magnetic layers have
antiparallel (AP) orientation either the first or the second layer restricts the
number of channels to 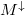 , and so the conductance for both spin
channels is
, and so the conductance for both spin
channels is 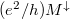 , i.e. the total conductance is
, i.e. the total conductance is
 . For parallel orientation of the magnetic
layers the conductance is
. For parallel orientation of the magnetic
layers the conductance is 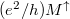 if the spin direction of the
carriers is the same as the magnetization, and it is
if the spin direction of the
carriers is the same as the magnetization, and it is 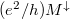 if
the magnetization has opposite orientation to the spin orientation of the
carriers. The net conductance is
if
the magnetization has opposite orientation to the spin orientation of the
carriers. The net conductance is
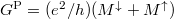 . The magnitude of the
magnetoresistance effect can be characterized by
. The magnitude of the
magnetoresistance effect can be characterized by 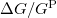 , where
, where
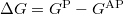 . With this notation the relative change of
the conductance turns out to be equal to the current spin polarization
characteristic to the ferromagnetic metal applied,
. With this notation the relative change of
the conductance turns out to be equal to the current spin polarization
characteristic to the ferromagnetic metal applied,
![\[ \frac{\Delta G}{G^\mathrm{P}}=\frac{M^\uparrow-M^\downarrow}{M^\uparrow+M^\downarrow}=P_c. \]](/images/math/8/a/a/8aae9d2087178d7af06b43665d07c925.png)
This simplified model corresponds to the perfectly ballistic limit, where no backscatterings are considered inside the quantum wire.
In a more realistic model the scattering inside the nanostructure should also be
considered. If the structure is smaller than the phase diffusion length,
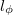 the scattering in the two layers are added coherently, and accordingly
the total resistance relies on the fine details of quantum interference
phenomena.
the scattering in the two layers are added coherently, and accordingly
the total resistance relies on the fine details of quantum interference
phenomena.
| 6. ábra |
\begin{figure} [!h]
\centering
\includegraphics[width=\columnwidth]{fig10.eps}
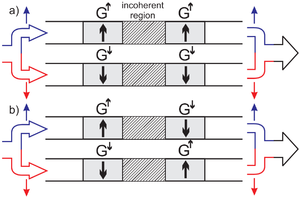
\caption{\it Resistive model of the GMR effect. The two magnetic layers are
separated by an incoherent nonmagnetic region. The top panel demonstrates the
parallel alignment of the magnetic layers (both layers have  magnetization direction), whereas the bottom panel demonstrates the
antiparallel alignment (the left layer has
magnetization direction), whereas the bottom panel demonstrates the
antiparallel alignment (the left layer has  and the right layer has
and the right layer has
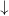 magnetization direction).
magnetization direction). 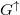 and
and 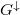 denote
the conductance of a layer for electrons with majority and minority spin
direction, respectively.}
\label{GMR2}
\end{figure}
denote
the conductance of a layer for electrons with majority and minority spin
direction, respectively.}
\label{GMR2}
\end{figure}
For even larger junctions, where phase coherence between the two magnetic layers
is already lost (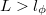 ), but the spin information is still preserved
(
), but the spin information is still preserved
(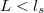 ), the two scattering regions are connected \emph{incoherently}
(Fig.~\ref{GMR2}), and accordingly their resistances are simply summed to get
the total resistance.\cite{note1} In this limit the magnetoresistance of the
spin valve structure is also easily calculated. To compare with the previous
ballistic model we calculate with conductances: the conductance for majority
spin states is
), the two scattering regions are connected \emph{incoherently}
(Fig.~\ref{GMR2}), and accordingly their resistances are simply summed to get
the total resistance.\cite{note1} In this limit the magnetoresistance of the
spin valve structure is also easily calculated. To compare with the previous
ballistic model we calculate with conductances: the conductance for majority
spin states is 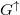 , whereas for minority spins it is
, whereas for minority spins it is 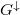 .
For antiparallel oriented magnetic layers the total conductance is
.
For antiparallel oriented magnetic layers the total conductance is
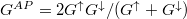 , whereas for
parallel oriented layers it is
, whereas for
parallel oriented layers it is 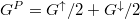 . From these
. From these
![\[ \frac{\Delta G}{G^\mathrm{P}}=\left(\frac{G^\uparrow-G^\downarrow}{G^\uparrow+G^\downarrow}\right)^2=P_c^2 \]](/images/math/1/9/3/193c43bfdda2554c0ee0f1ba2ac691a3.png)
follows. Note, that this model is equivalent with the common resistor model of the GMR phenomenon.\cite{0022-3727-35-18-201}
Both of the above simple models show that 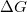 is positive, i.e. the
parallel orientation has higher conductance than the antiparallel one. Depending
on the model, the relative conductance change ranges between
is positive, i.e. the
parallel orientation has higher conductance than the antiparallel one. Depending
on the model, the relative conductance change ranges between 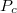 and
and 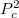 which indicates that a more complicated model based on the coherent
superposition of two scattering regions would also give a result between the
above two extreme limits. These considerations show that for typical values of
which indicates that a more complicated model based on the coherent
superposition of two scattering regions would also give a result between the
above two extreme limits. These considerations show that for typical values of
 , the relative amplitude of the GMR effect is expected to be
significant regardless of the details of the model. Note, however, that this
is only valid if the spin information is fully preserved in the spacer layer. As
the separation of the magnetic layers becomes larger than the spin diffusion length the GMR
effect exponentially decays.\cite{PhysRevB.48.7099,0953-8984-19-18-183201}
, the relative amplitude of the GMR effect is expected to be
significant regardless of the details of the model. Note, however, that this
is only valid if the spin information is fully preserved in the spacer layer. As
the separation of the magnetic layers becomes larger than the spin diffusion length the GMR
effect exponentially decays.\cite{PhysRevB.48.7099,0953-8984-19-18-183201}
The magnetic layers of a spin valve are decoupled by paramagnetic spacer, and this allows switching between parallel and antiparallel alignments. As the relative alignment depends on the external magnetic field, the GMR effect can be used for magnetic sensing. For this purpose the orientation of one layer is pinned by growing it on top of an antiferromagnet, while the unpinned free layer can rotate even under the influence of a weak magnetic field. Spin valves are extensively applied as the read head of hard disks, which utilizes the fast and reliable reading of the magnetic information by an electric signal.
The spin valve structure can also be used to store magnetic information. This type of non-volatile memory consists of a large number of spin valves, each of them being addressed separately in a crossbar wire architecture. The orientation of the free layer defines bit ``0 and bit ``1, which can be changed by the stray field of the nearby crossing wires. The information written in this way is red out by the GMR effect. However, the areal density of this type of magnetoresistive random access memories (MRAM) is limited by the length scale of the slowly decreasing stray fields.
In the most advanced novel MRAMs no external field (and extra wiring) is required to write information in a spin valve memory element. In these devices the orientation of the free layer is manipulated by high density spin polarized currents, while the magnetic information is obtained by low current GMR measurements. The writing is based on the spin-flip electron scattering processes occurring in the free layer, which exert a torque on the magnetization. Even though such spin-flips are rare events (the spin diffusion length is much larger than the characteristic size of the nanodomain), at current densities of about \(10^9 -10^{10}\, \textrm{A/cm}^2\), the induced torque becomes large enough to reverse the magnetization. The details of the spin transfer torque phenomenon are reviewed in Ref.~\cite{Ralph20081190}. Here we just point out the importance of the strongly non-equilibrium state of this nanoscale device: while the above current densities would lead to melting in any macroscopic metal, in this device the length scales which determine its transparency (i.e. its resistance) are well below the inelastic mean free path, and as a consequence the Joule heat is produced outside the device, in a much larger volume. In spite of the huge current densities, the nanoscale electronics defines appropriate signal levels for practical applications (below \(100\,\mu\)A, and around \(1\,\)V). Finally it is to be noted that the spin transfer torque magnetoresistive random access memory (STT-MRAM) is one of the most promising candidate for future spintronic applications.
\section{Conclusions}
Nanoscale phenomena of spin related transport were discussed both theoretically and experimentally. Based on the spin dependent band structure of a magnetic metal, we discussed the propagation of electrons in the ballistic and diffusive limits, and by applying the Landauer formalism, we supplied a \emph{nanoscopic} background for the conventional expression of the current spin polarization. As the most direct experimental method for spin polarization measurements, the Andreev reflection spectroscopy was introduced, and experimental results on Fe and Co were shown. The analysis demonstrated the reliability of measurements carried out in the ballistic limit. The method was also extended for the determination of the spin diffusion length, one of the most important parameters of metals in spintronic applications. The operation of spin valves was described in terms of the Landauer formalism and the magnitude of the GMR effect was determined in the coherent ballistic and the incoherent diffusive limits. Finally a short overview on spin valve applications was given.