Integrálás - Területszámítás
A Fizipedia wikiből
A lap korábbi változatát látod, amilyen Gombkoto (vitalap | szerkesztései) 2013. május 31., 11:04-kor történt szerkesztése után volt.
| [rejt] Navigáció Pt·1·2·3 |
|---|
| Kísérleti fizika gyakorlat 1. |
| Gyakorlatok listája: |
| Integrálás |
| Feladatok listája: |
| © 2012-2013 BME-TTK, TÁMOP4.1.2.A/1-11/0064 |
Feladat
- Határozzuk meg az
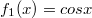 és az
és az 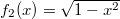 függvéynek által közrefogott területet a [-1,1] intervallumon!
függvéynek által közrefogott területet a [-1,1] intervallumon!
Megoldás
A keresett területet a![\[T =\left| \int\limits _{-1} ^1 (f_1 (x) - f_2 (x) dx \right| \]](/images/math/9/8/e/98ef683cb104be5255236e0b596edeca.png)
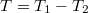 . Másrészt az
. Másrészt az 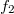 görbe egy egység sugarú félkör, tehát
görbe egy egység sugarú félkör, tehát 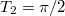 -t kell kapjunk.
Az 1. függvény primitívfüggvénye
-t kell kapjunk.
Az 1. függvény primitívfüggvénye 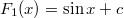 , így
, így 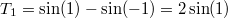 .
. 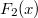 meghatározásáhzo végezzük el az
meghatározásáhzo végezzük el az 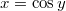 helyettesítést:
helyettesítést: ![\[f_2 (y) = \sqrt{1- \cos^2 y} = |\sin y |,\]](/images/math/5/e/6/5e6ddf870f71754aec4897a3a3493eb1.png)
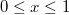 intervallumon integrálunk, akkor
intervallumon integrálunk, akkor 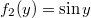 , továbbá
, továbbá ![\[\frac {dx}{dy} = -\sin y,\]](/images/math/4/d/3/4d39cd8cb014d7fd1cfdc44d4a2519cf.png)
![\[dx= -\sin y dy ,\]](/images/math/7/d/9/7d9bf718793591854798c78556e9857f.png)
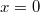 -nál
-nál 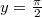 és
és 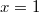 -nél
-nél 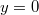 , ezzel
, ezzel ![\[\int\limits_ { x = 0} ^{ x = 1 } f_2 (x) dx = -\int\limits_ {y = \frac{ \pi}{2} } ^{ y = 0} \sin^2 y dy = \int\limits_ { \frac{ \pi}{2}} ^0 \left(\frac{\cos 2 y - 1}{2} \right) dy = \left[ \frac{\sin2y}{4} - \frac{y}{2} \right] _{ \frac{ \pi}{2}} ^0=0 + \frac { \pi }{4},\]](/images/math/8/a/4/8a446723658d6537aeac7a1decb7df27.png)
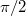 . Végül a keresett terület
. Végül a keresett terület ![\[T=2sin(1)-\frac{\pi}{2}\]](/images/math/4/8/c/48cf96de041ae005286cac93dbc7f5ca.png)